test development
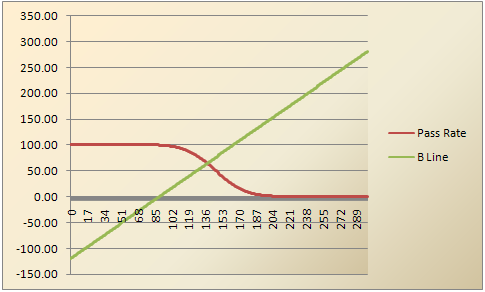
Estudio del método Angoff modificado
Un estudio con el método Angoff modificado es una de las formas más comunes de establecer una puntuación de corte defendible en un examen. Por lo tanto, significa que las decisiones de aprobado/reprobado tomadas por

Modified-Angoff Method Study
A modified-Angoff method study is one of the most common ways to set a defensible cutscore on an exam. It therefore means that the pass/fail decisions made by the test are more trustworthy than if

Item Writing: Tips for Authoring Test Questions
Item writing (aka item authoring) is a science as well as an art, and if you have done it, you know just how challenging it can be! You are experts at what you do, and

What is Item Banking? What are Item Banks?
Item banking refers to the purposeful creation of a database of assessment items to serve as a central repository of all test content, improving efficiency and quality. The term item refers to what many call
